The prisoners dilemma game is used as a model or at least a metaphor, for addressing different types of public goods problems and social dilemmas. In its earliest appearance it was formulated for experimental purposes. In January 1950, Dresher and Flood at the Rand Corporation conducted and experiment which seems to have introduced the Prisoner?s Dilemma.

The game refers to an imaginary situation in which two individuals are arrested and accused of having cooperated to commit some crime. The two prisoners are held separately, and attempts are made to induce each one to implicate the other. Each prosoner will receive a relatively light sentence (a relatively good payoffs) if both prisoners choose to cooperate with each other and don't confess. But if both prisoners choose not to cooperate with each other, and confess, then both prisoners receive a relatively heavy sentence (a relatively bad payoff). What does game theory predict will happen? Click here for an introductory explanation of the Prisoner's Dilemma game and the game theory prediction.

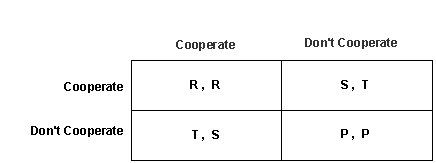
A general symmetric Prisoner?s Dilemma (PD) game setup would be as follows

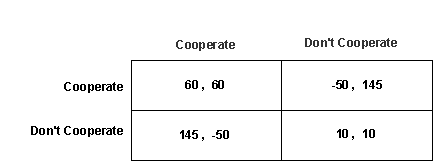
Such that T>R>P>S, and for repeated play (T+S<2R) A particular example used in experiments by Selten and Stocker(1986).

(Don't Cooperate,Don't Cooperate) in the general formulation is the dominant strategy unique Nash Equilibrium of the one shot game. However, in a repeated game, where players play more than once, a strategy would be a function of the history of the game up to k periods. There have been different strategies observed, like Tit for Tat, Grim strategy, and carrot and stick strategies.

One of the well known experiments involving the prisoner?s dilemma is by Axelrod ( Axelrod and Hamilton, 1981). Robert Axelrod was interested in finding a winning strategy for repeated prisoner's dilemmas games. He conducted a computer tournament where people were invited to submit strategies for playing 200 games of prisoner's dilemma (Axlerod and Hamilton, 1981). Fourteen game theorists in disciplines such as economics and mathematics submitted entries. These 14, and a totally random strategy, were paired with each other in a round robin tournament. Some of these strategies were highly intricate. But the result of the tournament was that the simplest of all strategies submitted attained the highest average score. This strategy, called TIT FOR TAT by its submitter Anatol Rapoport, had only two rules. On the first move, choose co-operate. On each succeeding move do what your opponent did the previous move. Thus, TIT FOR TAT was a strategy of cooperation based on reciprocity ( For a discussion of concepts such as Trust and reciprocity click here.

Another major strand of research studies learning behaviors, and in particular end game behavior in finite Prisoner?s Dilemma supergames. Pioneers in this area (Selten and Stoecker,1986) proposed a learning theory which models the experience of end game behavior in finite Prisoners Dilemma supergames. The theory was compared with the experimental results. In their experiment, 35 subjects participated in 25 Prisoner?s Dilemma supergames of ten periods each against anonymous opponents, changing from supergame to supergame. The typical behavior of experienced subjects involved cooperation until shortly before the end of the supergame.

The pattern of cooperation followed by an end-effect where people defect is observed in almost all super games between experienced players. Straightforward game theoretical reasoning cannot explain experienced behavior in finite prisoner?s dilemma supergames. One could try to account for this by the assumption that the players? utility is different from the monetary rewards. Players for example may value cooperation as such and therefore refrain from non-cooperative behaviors in spite of monetary effects. However, such explanations fail to be convincing in view of the end effects where the monetary incentives become stronger than the desire to be cooperative. An attempt to explain cooperation followed by an end effect as the result of fully rational behavior may be based on the idea of slightly incomplete information on the other players payoffs (Kreps, Milogram, Roberts and Wilson,1982).

Selten and Stoecker prisoner's dilemma

1. Selten, Reinhard and Stoecker (1986)?End Behaviour in Sequences of Finite Prisoner?s Dilemma Supergames? A Learning Theory Approach,? Journal of Economic Behaviour and Organisation, 7, 47-70
2. Kreps, Milgram, Wilson (1982) "Rational Cooperation in the finitely repeated Prisoner?s Dilemma," Journal of Economic Theory 27, 245-252
3. Axelrod (1984). The Evolution of cooperation (Basic Books, New York).